In the process of exploring an object, it is often impractical or even impossible to deal directly with that object. It is more convenient to replace it with another object similar to this one in those aspects that are important in this study. In general, a model can be defined as a conditional image (simplified image) of a real object (process), which is created for a deeper study of reality. The method of research based on the development and use of models is called modeling. For example, a model of an airplane is blown in a wind tunnel, instead of testing a real airplane – it is cheaper. In the theoretical study of the atomic nucleus, physicists present it in the form of a liquid drop that has a surface tension, viscosity, etc. The need for modeling is due to the complexity, and sometimes the impossibility of direct study of a real object (process). It is much more accessible to create and study prototypes of real objects (processes), i.e. models. to say that theoretical knowledge of something is usually a collection of different models. These models reflect the essential properties of a real object (process), although in fact reality is much more substantial and richer.
A model is a mentally represented or materially realized system that, by displaying or reproducing an object of study, is able to replace it so that its study gives new information about this object.
The cognitive capabilities of the model are determined by the fact that the model reflects any essential features of the original object. The question of the necessity and sufficient similarity of the original and the model requires specific analysis. Obviously, the model loses its meaning both in the case of identity with the original (then it ceases to be the original), and in the case of excessive difference from the original in all significant respects.
Thus, the study of some sides of the simulated object is carried out at the cost of refusing to reflect other parties. Therefore, any model replaces the original only in a strictly limited sense. From this it follows that for one object several “specialized” models can be built, concentrating attention on certain aspects of the object under study or characterizing the object with varying degrees of detail.
The similarity between the simulated object and the model can be physical, structural, functional, dynamic, probabilistic and geometric. In physical similarity, the object and the model have the same or similar physical nature. Structural similarity implies the existence of similarities between the structure of the object and the structure of the model. When an object and a model execute similar functions under certain influences, a functional similarity is observed. When observing the successively changing states of the object and the model, dynamic similarity is noted. Probabilistic similarity is noted when there is a similarity between the processes of a probabilistic nature in the object and the model. Geometric similarity occurs when the spatial characteristics of the object and the model are similar.
To date, there is no universally accepted unified classification of models. However, from the many models, you can distinguish verbal, graphic, physical, economic-mathematical and some other types of models.
A verbal or monographic model is a verbal description of an object, phenomenon, or process. Very often it is expressed in the form of definitions, rules, theorems, laws or their totality.
A graphical model is created as a drawing, a geographic map, or a drawing. For example, the relationship between price and demand can be expressed in the form of a graph, on the axis of the ordinate, whose demand is deferred (D), and on the abscissa axis , the price (P). The curve clearly illustrates to us that as the price rises, demand falls, and vice versa. Of course, this dependence can be expressed verbally, but graphically it is much clearer (Fig. 1.1).
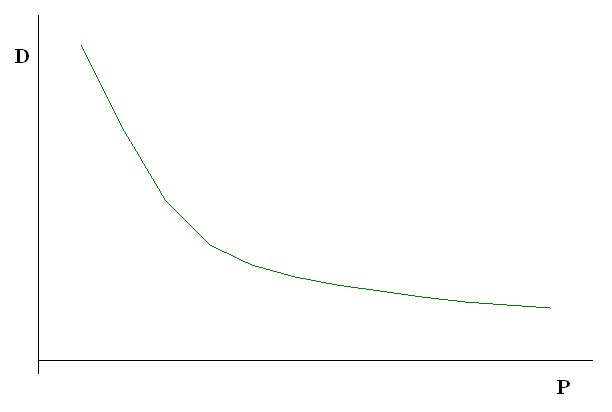
Rice. 1.1. Graphical model showing the dependence between demand and price
Physical or material models are created to construct objects that do not yet exist. Creating a model of an airplane or rocket to test its aerodynamic properties is much easier and more cost-effective than studying these properties on real objects.
When modeling, an analogy is used between the object – the original and its model. Analogies are as follows:
external analogy (model of an airplane, ship, microdistrict, pattern); structural analogy (the water supply network and the power grid are modeled using graphs that reflect all connections and intersections, but not the lengths of individual pipelines); dynamic analogy (in the behavior of the system) – the pendulum simulates an electrical oscillatory circuit.
Mathematical models belong to the second and third type. The meaning of mathematical modeling is that experiments are conducted not with a real physical model of an object, but with its description. They are characterized by the fact that they are implemented using information technology. The content of any economic-mathematical model is the economic essence of the conditions of the problem and the goal expressed in formal and mathematical relations. In a model, an economic quantity is represented by a mathematical relation, but not always a mathematical relation is an economic one. “The economic-mathematical model is a concentrated expression of the general interrelations and regularities of the economic phenomenon in a mathematical form” (academician V.S. Nemchinov).
Economic and mathematical models reflect the most essential properties of a real object or process using a system of equations. There is also no single classification of economic and mathematical models, although it is possible to distinguish the most significant groups of them depending on the feature of classification.
According to the degree of aggregation of modeling objects, models are distinguished:
microeconomic; one-, two-sector (one-, two-product); multisectoral (multi-product); macroeconomic; Global.
By taking into account the time factor, the models are divided into:
static; Dynamic.
In static models, an economic system is described in statics, in relation to one particular point in time. It’s like a snapshot, a slice, a fragment of a dynamical system at some point in time. Dynamic models describe an economic system in development.
According to the purpose of creation and application, models are distinguished:
balance sheets; econometric; optimization; network; queuing systems; imitation (expert).
Balance models reflect the requirement that resources be available and used.
The parameters of econometric models are estimated using the methods of mathematical statistics. The most common econometric models are systems of regression equations. These equations reflect the dependence of endogenous (dependent) variables on exogenous (independent) variables. This dependence is mainly expressed through the trend (long-term trend) of the main indicators of the simulated economic system. Econometric models are used to analyze and predict specific economic processes using real statistical information.
Optimization models make it possible to find the best option for production, distribution or consumption from a variety of possible (alternative) options. Limited resources will be used in the best way to achieve this goal.
Network models are most widely used in project management. The network model displays the complex of works (operations) and events and their interrelation in time. Typically, the network model is designed to perform work in such a sequence that the project deadlines are minimal. In this case, the task is to find a critical path. However, there are also such network models that are focused not on the time criterion, but, for example, on minimizing the cost of work.
Queuing models are created to minimize the time it takes to wait in line and the downtime of service channels.
The simulation model, along with machine decisions, contains blocks where decisions are made by a person (expert). Instead of direct human participation in decision-making, the knowledge base can act. In this case, a personal computer, specialized software, a database and a knowledge base form an expert system. The expert system is designed to solve one or a number of problems by imitating the actions of a person, an expert in this field.
According to the uncertainty factor, the models are divided into:
deterministic (with unambiguously defined results); stochastic (with different, probabilistic results).
According to the type of mathematical apparatus, models are distinguished:
linear and nonlinear programming; correlation-regression; matrix; network; game theory; queuing theory, etc.